Gregorian calendar 1601-4000

The Gregorian Calendar is currently the most widely useful civil calendar. It is named after Pope Gregory XIII, who introduced the calendar in 1582.

CALENDAR VS. TROPICAL YEAR VS. EQUINOX-TO-EQUINOX YEAR

It’s worth explaining the importance of the correlation between the calendar, the tropical year, and the equinox-to-equinox year. The Gregorian calendar is the successful successor of the Julian Calendar, in which the whole period exceeds the tropical year by about 11 minutes. As a result, the accumulated error of one day is observed after just 128 years.
Comparing the Gregorian Calendar to the Julian one it’s practically errorless. It encloses a period of 400 years, which includes exactly 97 leap years and 303 ordinary years. The leap year occurs every 4 years. The exception is years exactly divisible by 100 unless they are divisible by 400 at once. It means, that the leap years are successively 1600, 2000,2400, etc whereas the years like 1700,1800, or 1900 are not.
The mean length of the Gregorian Calendar is 365.2425 days, which is longer than the tropical year by just 0.00031 days. It results in an accumulated error of one day only after 3200 years, but it’s just far-reaching simplification. This is because the length of the tropical year is not strictly constant. It is slowly decreasing by about half a second per century. The accumulated effect is proportional to the square of the time. It means, that when the first year will be shorter by 0.005 seconds, the second one will be shorter by 0.001 seconds. The total error at the end of the second year will be 0.015.
Considering 10000 years, the difference will amount to:

(1+2+3+....+10000) x 0.005 seconds

accumulating the addition error to the value of 250000 seconds. Since one day counts 86400 seconds, this difference will be about 2,89 days.
On the other hand, we cannot rely on this pace of decrement of the tropical year, because it’s driven by the precession of Earth’s rotation axis. The length of the tropical year is not decreasing uniformly with time and nowadays (years 2000+) is the fastest, reaching -0.532 seconds per century.
The tropical year is defined as the period needed Sun’s mean longitude to increase by 360º. This period is slightly different from the equinox-to-equinox year. The length of the equinox-to-equinox year is driven by changes in the tropical longitude of the perihelion, which is measured from the vernal equinox of the date. It retrogrades as the effect of the precession of Earth’s rotation axis by 50″.3.  Let’s assume, that the Sun is at the spring equinox. One year later the Sun has made a complete circuit analog to the ecliptic and has returned to the spring equinox point, whereas the Earth hasn’t yet as the perihelion point measured from the vernal equinox of the date has moved over 50″3. So we can see, that the equinox-to-equinox year is slightly shorter than the tropic year. As a matter of fact, after a considerably more extended period, the length of astronomical seasons will change the same as the length of the year as measured from the spring equinox to another spring equinox. The basic aim of the Gregorian Calendar is to keep the spring equinox date as close as possible to March 21. In turn, we cannot use the tropical year to measure the accuracy of the Gregorian Calendar.

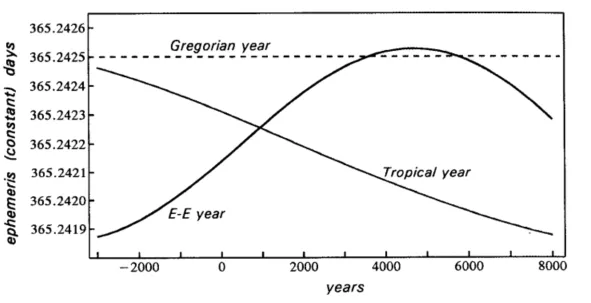
Between the years AD 3400 and AD 6000, the length of the eEquinox-to-equinox year is very close to the Gregorian year, which results in an almost constant time of the equinox. Therefore we have clear proof, that the commonly assumed 1-day error after 3200 years isn’t working, because, in reality, the difference is much less. Between AD 2000 and AD 8000, the variation will be just about 1/4 day!
Another factor is the Earth’s rotation, which gradually slows down resulting in the lengthening of the day by 0.001 seconds after one century. In turn, the picture above might not be valid 100%, although even with this factor, the Gregorian calendar will not be more than one day off (as compared to AD 2000) until about AD 7200. We can assume, that the Gregorian calendar is an excellent solution up to the next 5 thousand years or so. Unfortunately, the slowing down of the Earth’s rotation will force another calendar reform. For example, after 2,4 million years from now, the length of the year will be only 365,1 days, so the leap year will be required just one per decade. We have indeed an evident conclusion, that there are no calendar rules valid forever.

 

THE WEEKDAY OF CHRISTMAS DAY

Depending on the length of the considered period, we can estimate the frequency of the Christmas Day occurrence on the given day of the week. Since in 28 year period, this day falls 4 times on each of the seven days of the week, the situation looks slightly different for longer periods. The period of 28 years is a multiple of 4 (the cycle of bissextile years), the same as the total number of days within this period – 10227 is a multiple of 7.  This rule is valid only in the 28-year cycle without interruption, so effectively it can be applied for multiple 28-year cycles within a maximum of 2 centuries, assuming that there is no leap year in the middle. In fact, it wasn’t applied to Julian Calendar, because it simply wasn’t needed.
Every year divided by 100 but not by 400 will perturb the game and result in the Christmas day occurrence somewhat on the “weekday before” (instead of Wednesday it will be on Tuesday).
Concluding the 400-year period, we can see the following Christmas Day occurrence:
56 times on Monday and Saturday,
57 times on Wednesday and Thursday
58 times on Tuesday, Friday, and Sunday

What is most interesting, is that similar results can be obtained for any other dates in the year. Following J.Meeus and bringing 4^th July as an example, we will see, that it occurs most often on Monday, Wednesday, or Saturday.
To receive correct results, the multiple of 400 years period must be always considered, because in the Gregorian Calendar, the periodicity of weekdays is exactly 400 years.

EASTER DATE

In the Julian Calendar, the periodicity of the Easter Date was 532 years. It worked on the same basis as the 400-year cycle of the Gregorian Calendar. The Easter Sundays are repeated exactly in the same order after the 532-year period. The two extreme possible Easter dates (22.03 and 25.04) were observed four times each in this period, giving the mean frequency of each time equal to 133 years. If we consider the slightly less extreme dates like March 23, 24, or April 23, 24, the frequency is twice higher (66,5 years). The most frequent Easter days in the Julian Calendar (532 years cycle) were: March 28, March 31, April 3, April 5, April 6, April 8, April 11, April 14, April 16, and April 19. The frequency of these particular dates was 26,6 years, as 20 occurrences in this cycle were observed.
In the Gregorian Calendar things are more tricky, as we cannot rely on the 532-year cycle anymore. In 3 out of 4 century years, the Gregorian leap-year rule causes a shift of 1 day forward in the date of the full Moon.

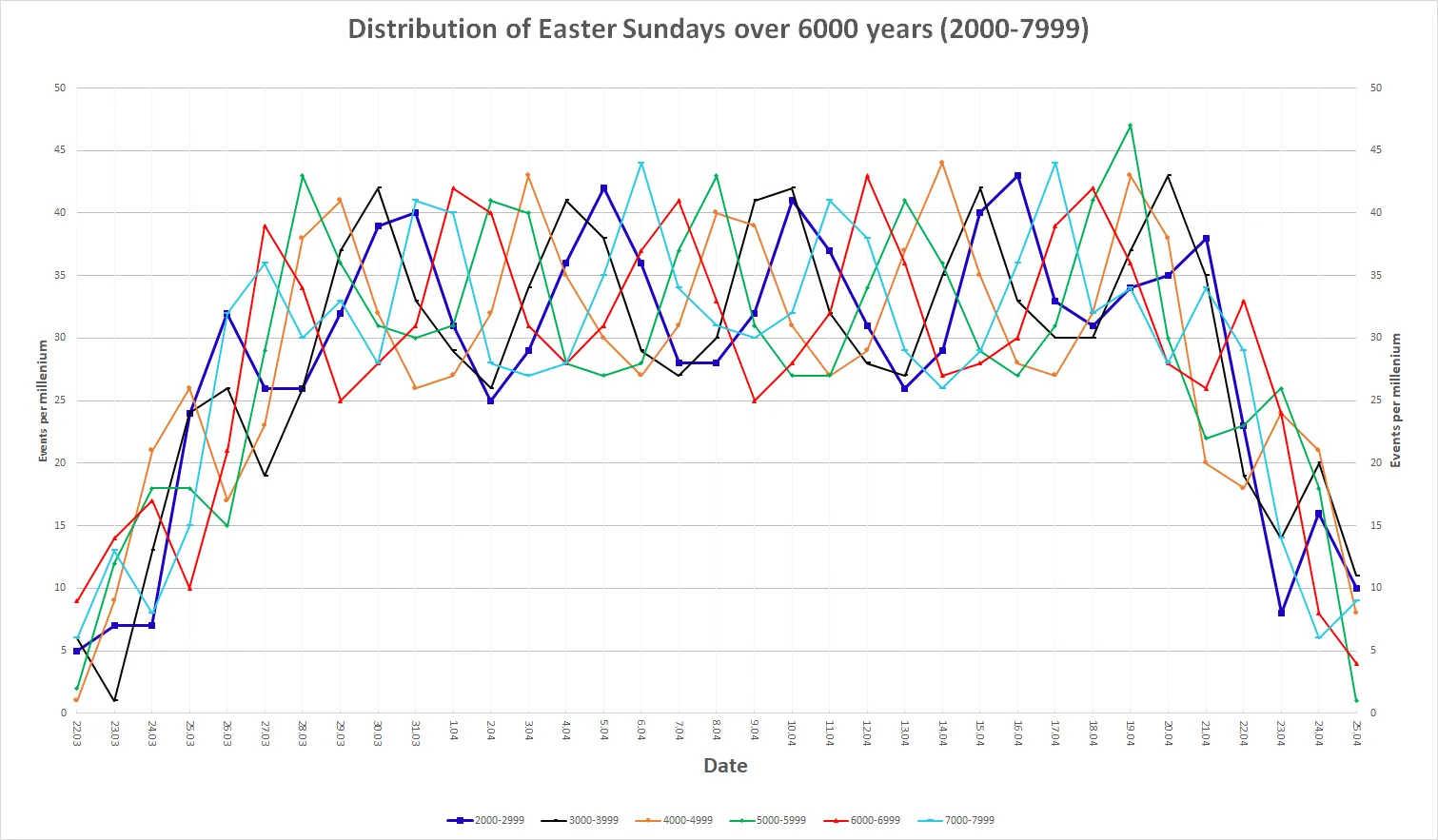
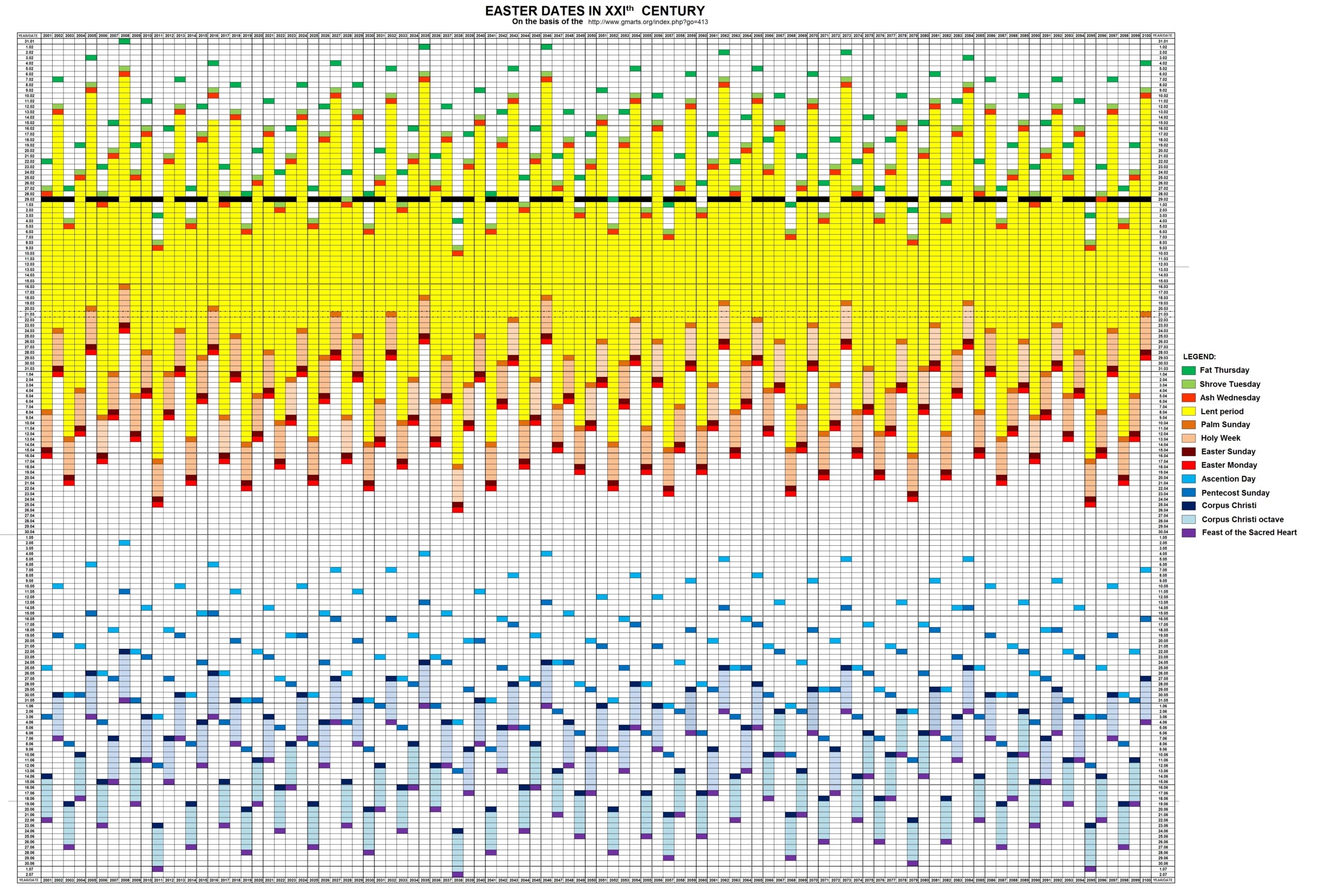
The image above shows the frequency of Easter Sundays between March 22 and April 25 in the span of 6000 years. This is the period, which at some point could be considered as the “small” Easter cycle in the Gregorian calendar. After 6000 years these maxima fall exactly on the same dates. However, it still doesn’t mean, that this period marks the full cyclical recurrence of the Easter dates. The full Easter cycle in the Gregorian Calendar is to be enclosed after 5700000 years! Indeed, it’s just a mathematical assumption, as this calendar won’t be valid until this time because of the changing motions of the Sun and Moon.
Interesting is the fact, that the most often Easter Sunday date over these 6000 years is April 19 (Pic. 3).

In trying to explain this phenomenon, we must take a look at the distribution of epacts in the Gregorian Calendar.
The exact period can have a twofold meaning. It can define the age of phases of the Moon in days on 22 March in the Gregorian Calendar, but it can also define the age of the ecclesiastical Moon on 1 January. The common denominator of the two is measuring the Moon’s age at a particular date. The epact shows the principal difference in time between the solar year and the lunar year. To be precise the epact means the days, by which the solar year exceeds the lunar year.
The primary condition for Easter Sunday to occur is, that this day must fall directly after the first full Moon after the vernal equinox, called also the “ecclesiastical Easter Full Moon”. The time interval for this occurrence is only between March 21 and April 18 inclusively.  Multiplying the number of epacts by all days of the week we receive the period of 210 years, in which, as presented in the table below, the total number of epacts falling under a certain date can be seen.

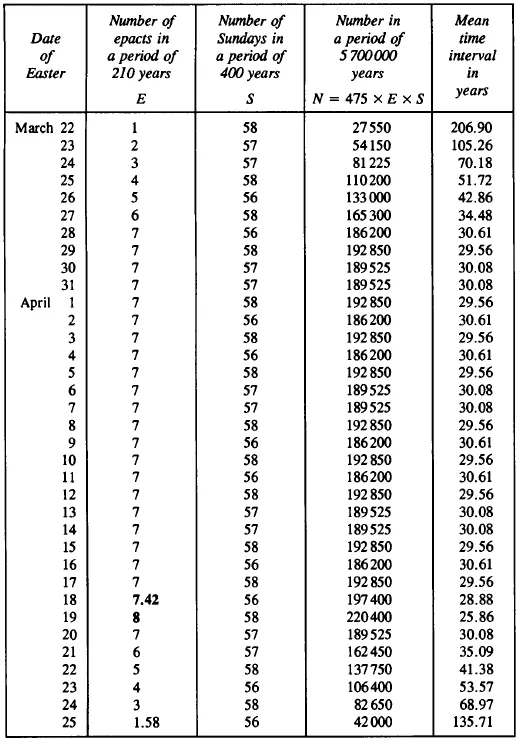
For example, if the Paschal Full Moon is observed on March 21, the possible Easter Sunday can occur from March 22 to March 28. The date of March 28 gives the opportunity for Easter Sunday occurrence somewhat in the place of 7 possible dates then, unlike March 22 with just one possible date.  Hence the 7 epacts can be seen in the 210-year period for most of the dates from March 28 to April 20. The situation changes slightly when approaching April 18 – the last date on which the first full Moon can be observed directly after the vernal equinox. Let’s see the situation when the number of epacts is higher than 7. Since it has been told, that the period of the Paschal Full Moon can be observed between March 21 and April 18. This period counts exactly 29 days, whereas the total number of possible epacts is 30 (0 to 29). Epact 0 means the new Moon in conjunction with the Sun, epact 1 represents the theoretical first visibility of the thin crescent of the Moon. Concluding, the particular number of epact corresponds to the age of the Moon. For instance, for Paschal Full Moon date of April 18, the epact number is 24, for the date of March 21, the epact number is 22, and so forth. So one of the epacts corresponds to somewhat 2 days. This is epact 25 falling somewhere between April 17 and April 18. In some sources, we can find the epact 24 assigned to April 17, but sometimes April 17 represents epact 26. In turn, we can see somewhat the accumulation of the possible epacts as one of the 7 different dates is doubled. This accumulation is observed effectively to April 25, the very last day, on which Easter Sunday is possible.
In the period of 5700000 years, when the Gregorian Calendar along with the distribution of Easter dates returns to exactly the same point, we can see that the most likely Easter Sunday date is April 19 (almost 4%), whereas the least is March 22 (about 0,5%). Interesting is another column in the table above, which states the number of Easter Sundays falling on a particular date in the 400 years Gregorian Calendar cycle. The respective values vary between 56 and 58, which was explained in the previous chapter. The next column shows the total number of Easter Sundays occurring at the given date in the period of 5700000 years. The April 19 Easter Sunday occurs 220400 times (231 times per 6000 years period), and the April 18 Easter Sunday is the second most frequent date and occurs 197400 times (208 times per 6000 years). Looking at the opposite side, the least frequent occurrence of Easter Sunday on March 22 indicates “only” 27550 events in the analyzed period (29 times per 6000 years) with a mean time interval of almost 207 years (rightmost column). It’s worth mentioning, that the last dates with Easter Sunday occurring on March 22 were 1693, 1761, and 1818. Next, after over 400 years of break, the dates will be 2285, 2353, and 2437. The second rarest Easter Sunday dates are observed on April 25. In the period of 5700000 years, it’s 42000 events (43 times per 6000 years). The Easter Sunday of April 25 can be observed mainly once in a given century. The last date was 1943, the next one is 2038, and the following one was 2190.
The last Easter date feature is their appearance. If we could take a look at the graph below (Pic. 5)…

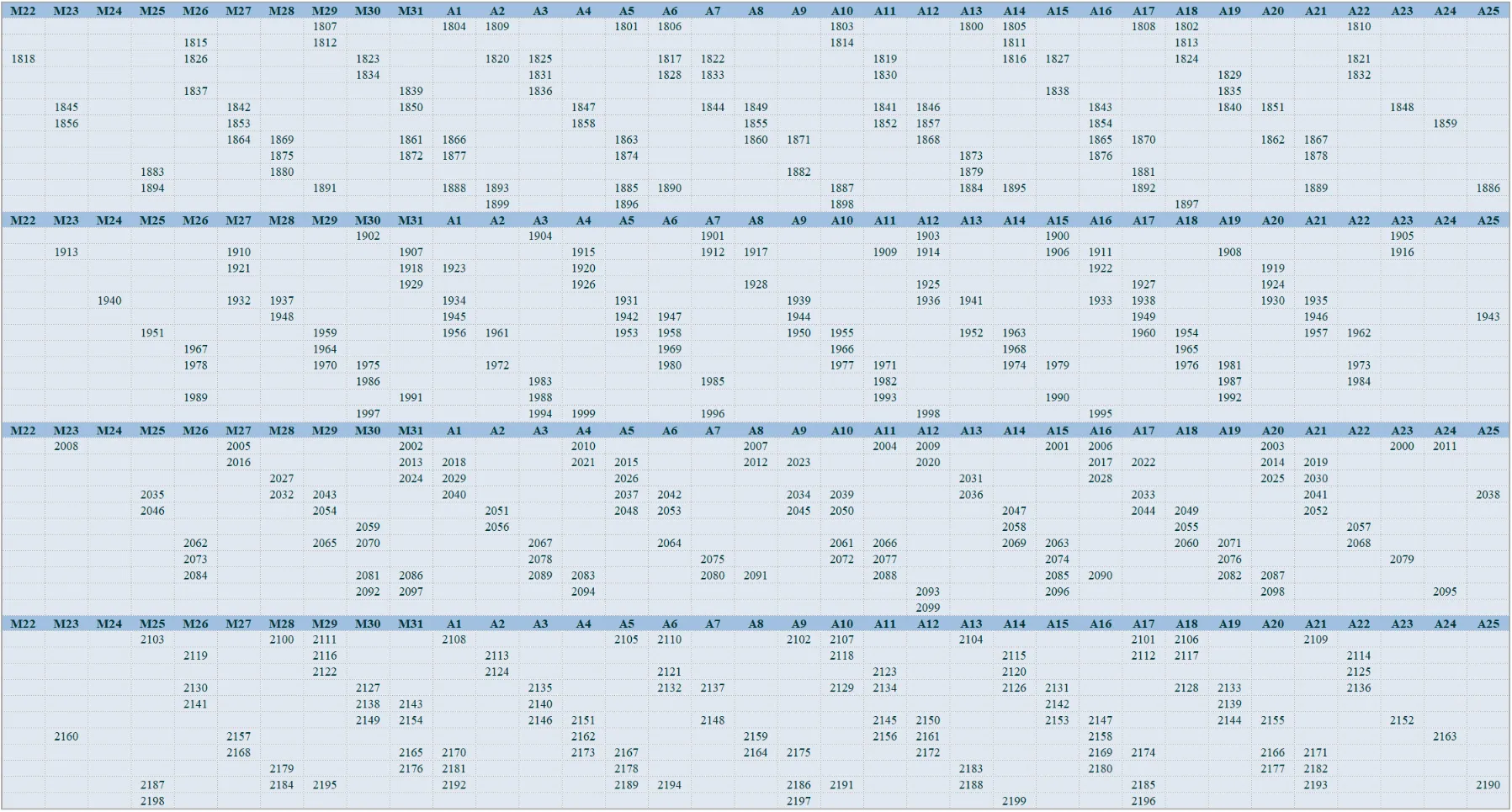
we can see, that they occur in series. These series can be analyzed by particular dates, as shown in the graph above (recurrences) as well as the years. Considering the second circumstance, for now, the good information can be that Easter Sundays, which occur in March are always isolated. It means, that there is no chance for successive Easter Sunday occurrences in March. The Easter date in March is always preceded and successes by the Easter date occurring in April. On the other hand, there are a few situations, where April’s occurrences of Easter Sundays are isolated too. The few known situations close to the present time are 1770,1781, 1838, 1990, 2085, and 2142.
Let’s take a look at an opposite situation when up to even 10 successive Easter dates can be observed in April. However the most curious is, that the combinations such as these have never taken place since the Gregorian Calendar is in use. They’re coming and the first one will be observed in the period 2856-2865. The series of 7 successive April Easters are more frequent, we have 47 cases in the period 1583-3000, but their distribution is also irregular. The current one is 2017-2023, the next one is 2036-2042, and the previous one is 1979-1985. Four series in the XX and XXI centuries, five in the XXII century, but just one in the XXIV and XXVI centuries.
Let’s back to the Easter recurrences, as presented in the table above (Pic. 5). The mechanism of the Gregorian Calendar allows the Easter Date to repeat on the same date after 11 years. The recurrence of Easter can include at most four of the same dates taking place at 11-year intervals, and then the last case always occurs in a leap year. Let’s analyze some of them, closest to the time when this text is produced:

1991-2002-2013-2024 - March 31
1995-2006-2017-2028 - April 16
2015-2026-2037-2048 - April 5
2019-2030-2041-2052 - April 21

Some group of Easter dates repeats after 152 years. The closest situation to ours will occur in the following period:
Years 1948 – 2047 -> Years 2100-2199 where:

1948 March 28 -> 2100 March 28
1949 April 17 -> 2101 April 17
1950 April 9  -> 2102 April 9
2047 April 14 -> 2199 April 14

but the total length of the group of years can include 48, 52, or 100 (Pic. 6).

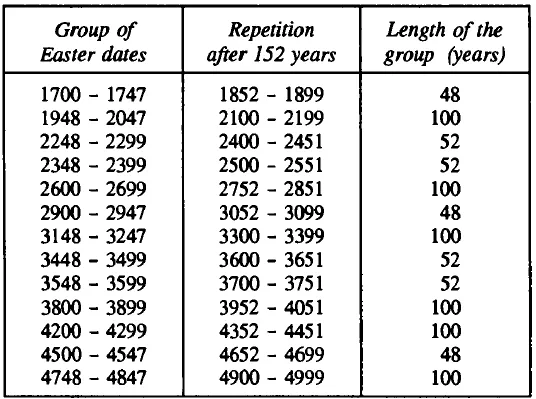
Finally, the repetition of a whole century happens after 5700000 years.

The last conundrum is the difference between the ecclesiastical and astronomical Easter. The ecclesiastical Easter relies on the Paschal full Moon, which is based on the mathematical approximations following the 19-year Metonic cycle. It was found, that the Paschal Full Moon is not strictly identical to the real Moon.  As a result, there are years when Easter Sunday occurs on an incorrect date from the astronomical point of view. In the astronomical sense, the spring equinox does not always occur on March 21 (Pic. 7)…

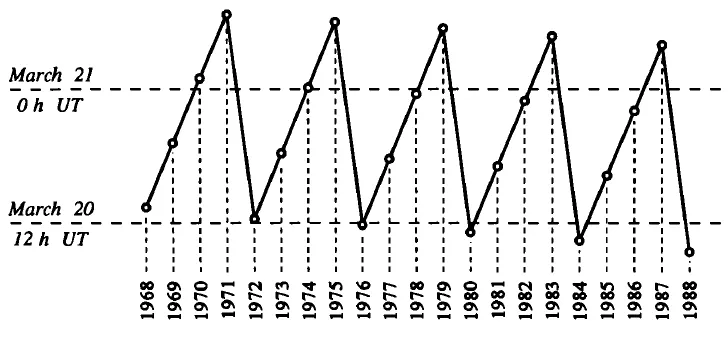
which means, that, the Paschal Full Moon does not exactly coincide with the full Moon. This is because the Christian church still follows the full Moon calendar, established in AD 325 which didn’t take the lunar motion as we know today.  The astronomical March equinox can vary between March 19 and even March 22, as the date depends on the timezone. In turn, the Easter date could occur even on March 21 (1666) or April 26 (1981, 2201), but it never happens. This is because the church date of the spring equinox is always March 21. In the span of 1000 years (1583-2582) we have 78 occurrences such as this.
The best example was in 2019, when the March equinox in the northern hemisphere occurred on March 20 at 21:58 UTC and the first full Moon afterward was on March 21 at 2:43 UTC. Despite the fact, that in many timezones it was already March 21, the ecclesiastical Easter was calculated from the next date of the full Moon, which occurred on April 18. It was like this, because if we would move our location several timezones back and bring the same example, to i.e. GMT-6, then we would have the March Equinox occurrence at 15:38 UTC-6 and a full Moon on the same day (March 20) at 20:43 UTC-6, hence effectively both events fall on March 20, which from ecclesiastical’s point of view is still not the spring equinox day!
The next example is the forthcoming 2038 year, when the spring equinox occurs on March 20 at 12:40 UTC and the full Moon can be observed immediately after – on March 21 at 2:09 UTC. Easter Sunday instead of March 28 shall take place only on April 25.
To have the given astronomical full Moon “accepted” as the Paschal full Moon, it must occur in the vast majority of time zones in the given day as long as they don’t fall under the next day. A good example was April 19, 1981, when the full Moon occurred at 7:59UTC exactly. All regions east of GMT-8 could see the full Moon on April 19, remaining timezones west of GMT-8, very late on April 18, but nowhere on April 20 (at GMT+14 the full Moon was observed at 9:59 pm), so the nearest Easter Sunday was on April 19.

The Christian Easter and Jewish Pash can coincide just 32 times in the period of 1583-3000. The last event like this occurred on April 19, 1981, and the next one will be on April 11, 2123.

---

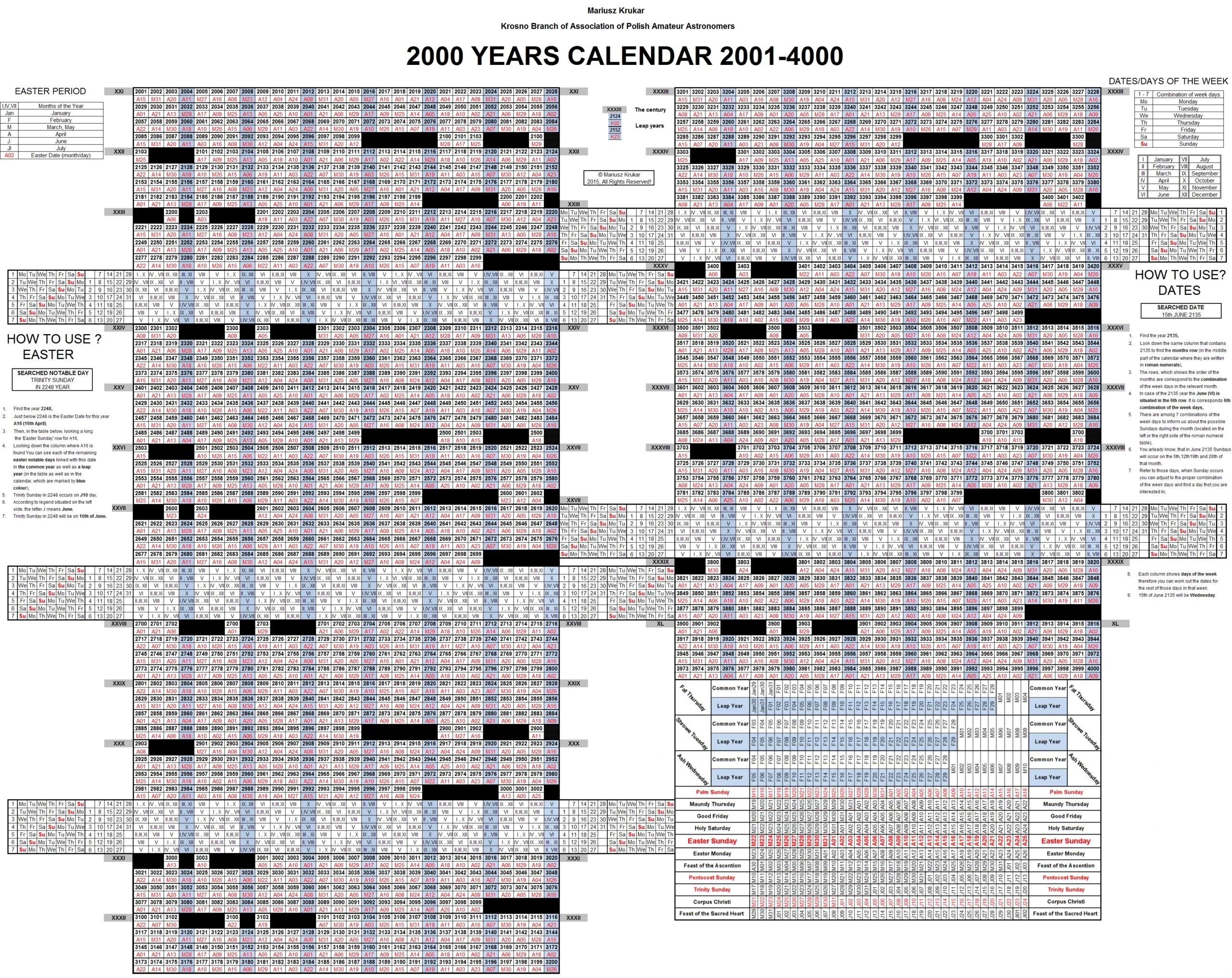
I prepared a few long-term calendars for the 1601-4000 period. I showed the way to compute the days and weeks also. All of my long-term calendars include easter dates. In order to use ready-prepared Easter dates I referred to The GM arts website, where Easter up to 4000 years is provided.

LONG TERM CALENDARS

50 years calendar 2016-2065 UK & Republic of Ireland

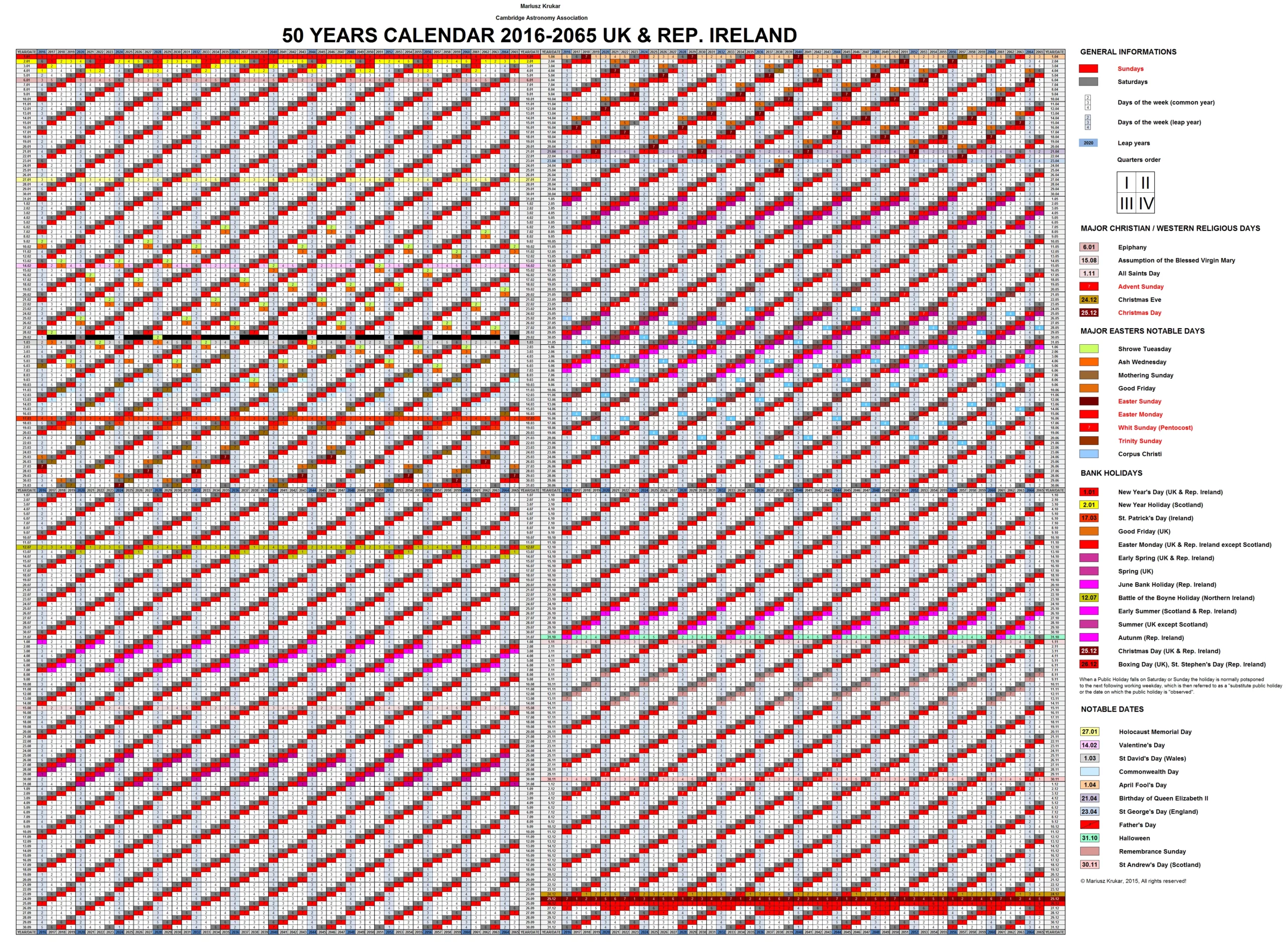
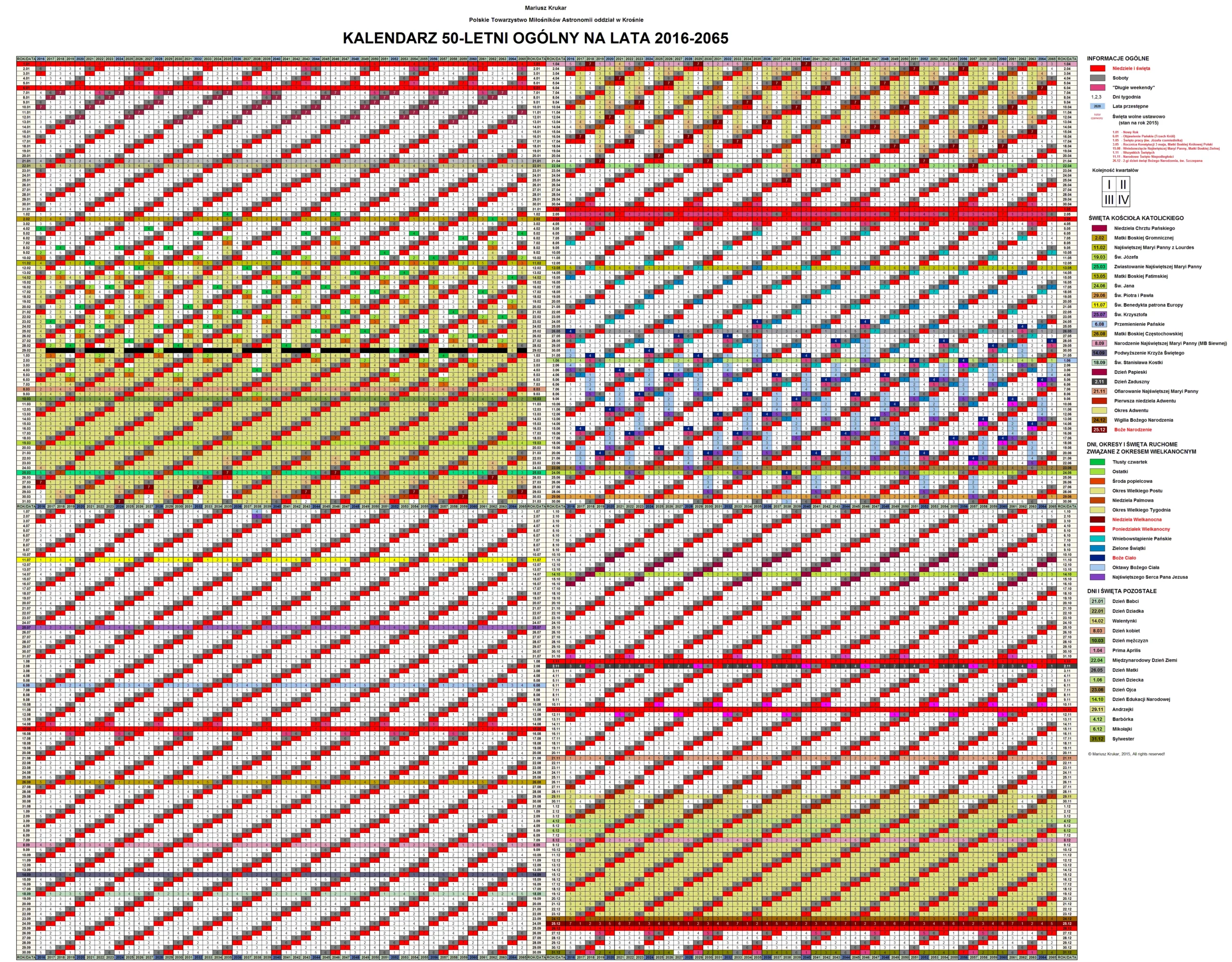
100 years calendar 2016-2115

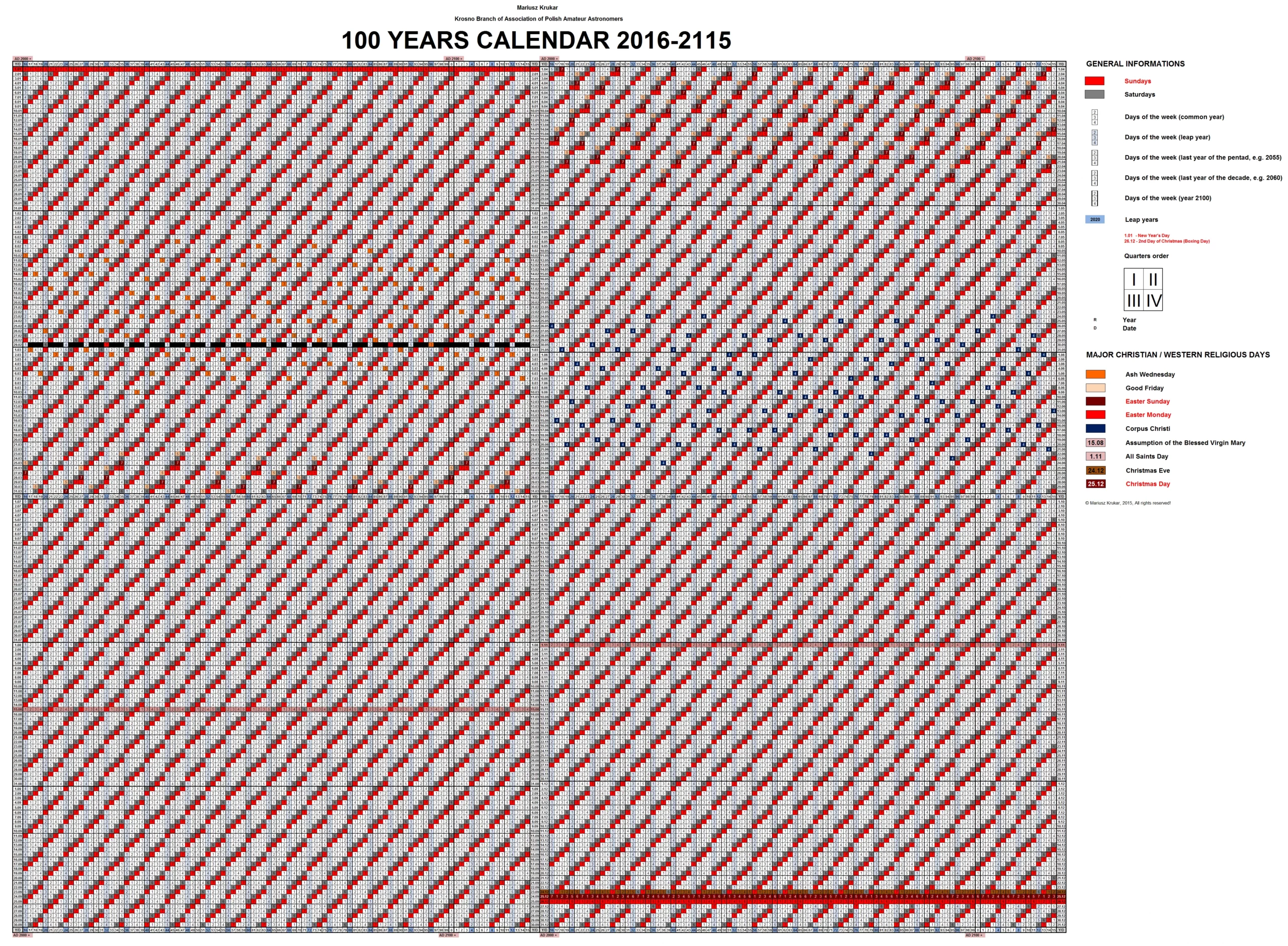
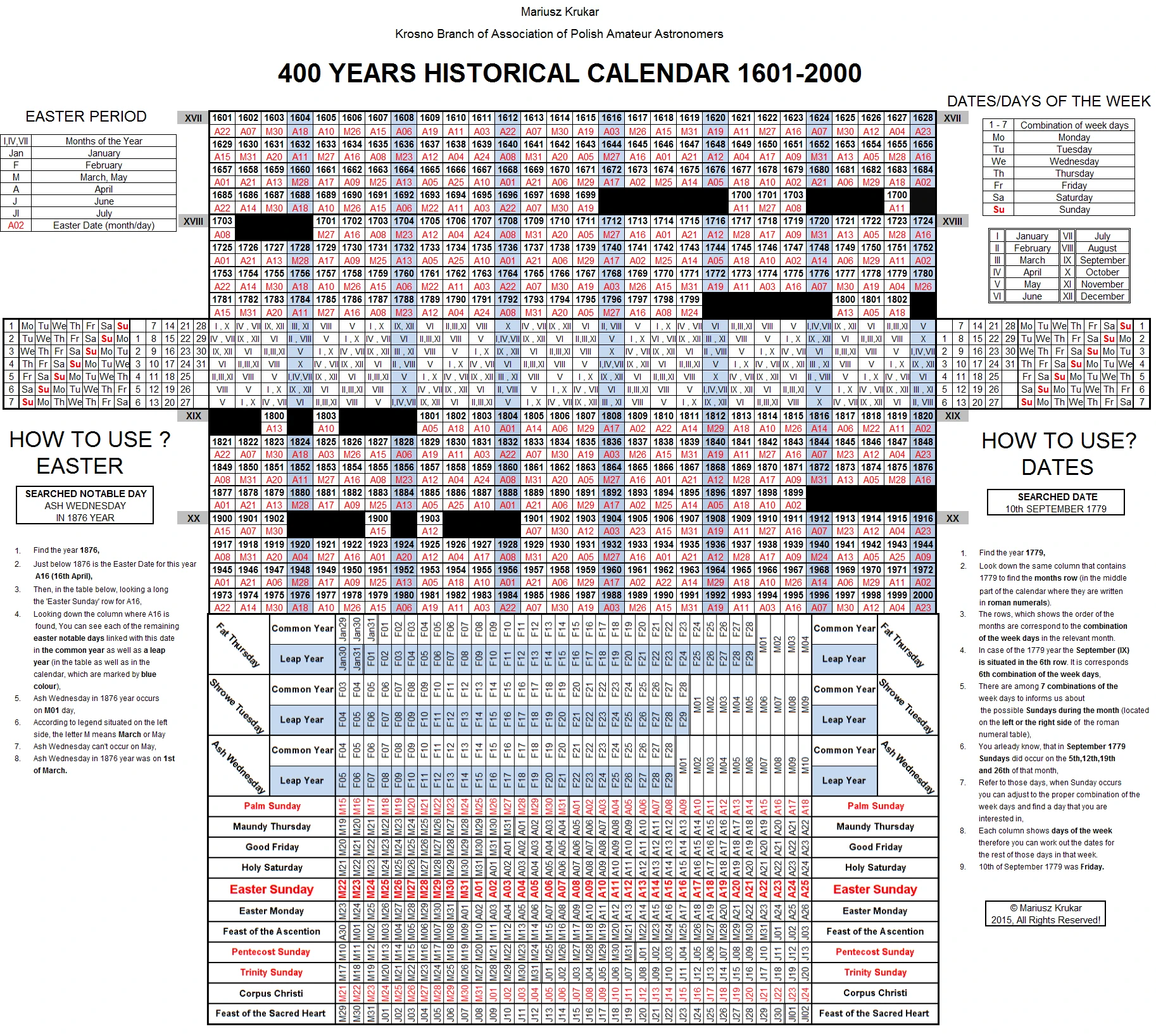
400 years historical calendar 1601-2000

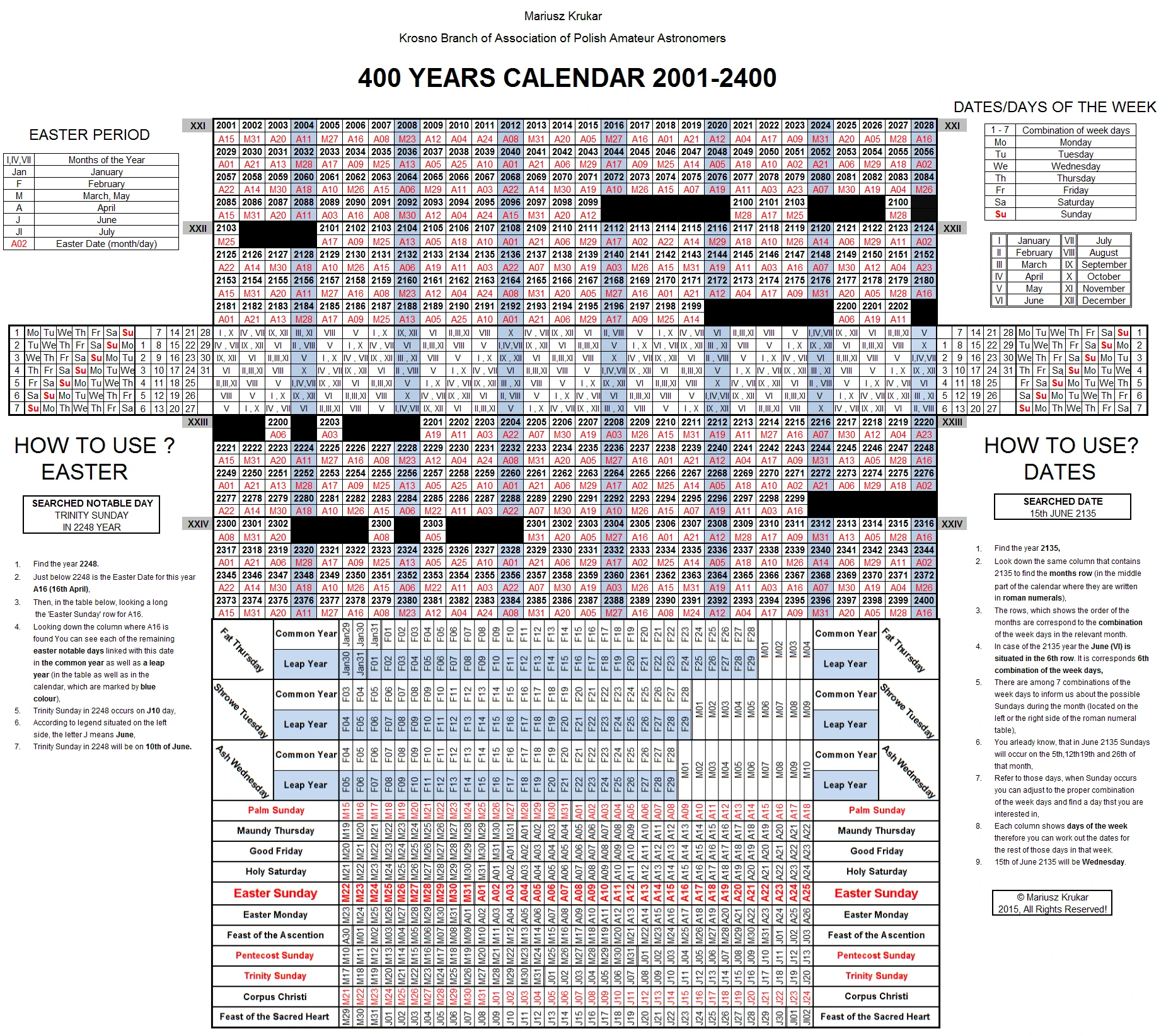
400 years calendar 2001-2400

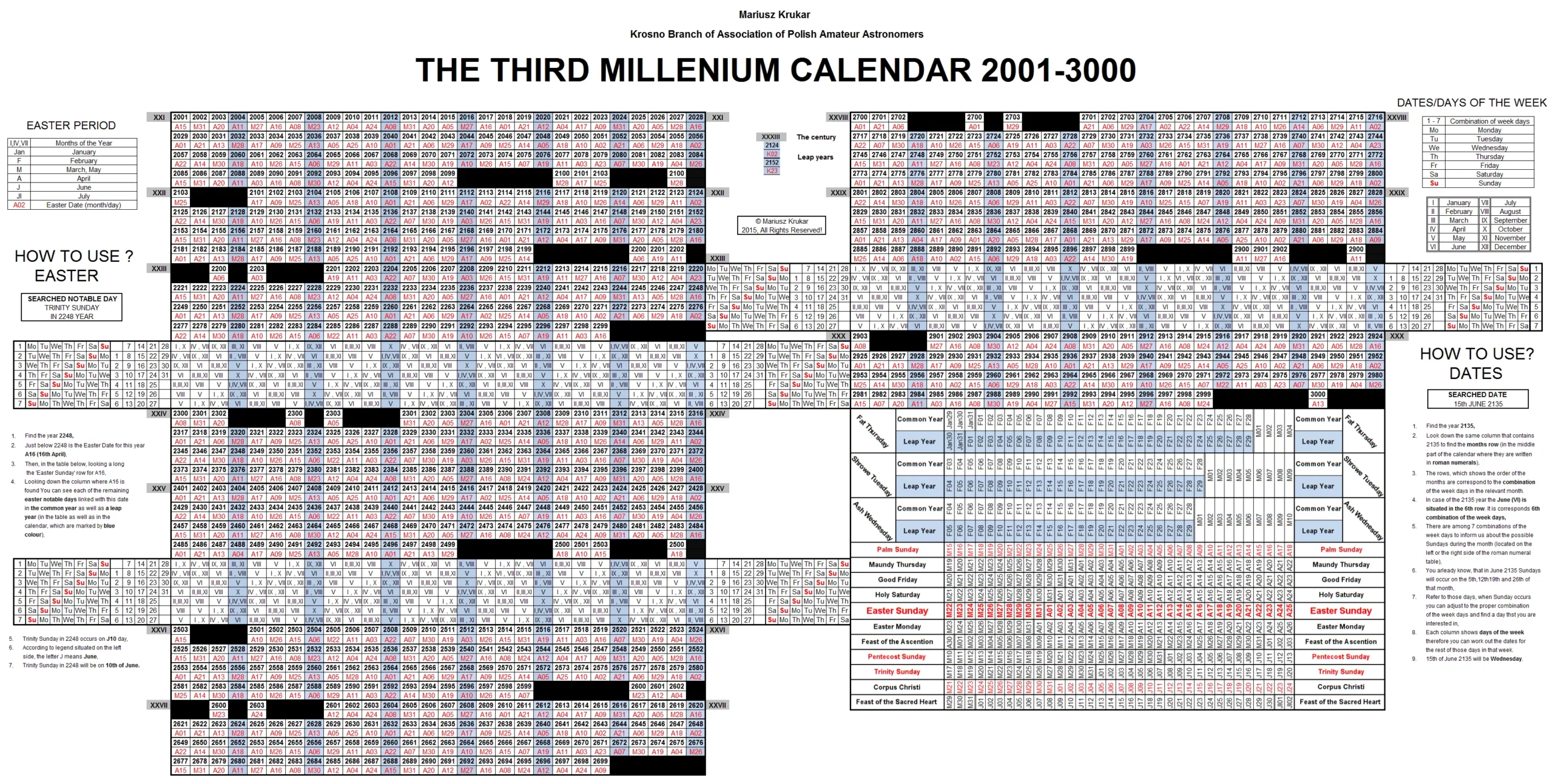
The Third Millenium calendar 2001-3000

2000 years calendar 2001-4000

VISIT THE SHOP AND BROWSE MORE

---

Apart from long-term calendars, I prepared a few useful tools, related to the Gregorian calendar mechanism, which you can visit at the links below:

1. The Easter Date 2001-2100 diagram – which helps you to find any holiday related to the Easter date. Simultaneously you can check your interesting date with respect to the Easter period.

2. The days of the week finder – gives you the opportunity to find:
– the day of the week for some interesting historical day, e.g. Constitution of May 3, 1791 (Tuesday),
– which day of the week were you born,
– find the day of the week for some interesting event in the future, e.g. Halley’s Comet perihelion on July 28, 2061 (Thursday).

3. The Gregorian calendar calculator – is shown in three major components:
– one year
– 100-200 years (period of all leap years i.e. 1901-2100)
– full Gregorian calendar cycle calculator

LEARN HOW TO USE IT

The 13^th Day

By examining the 400-year period of the Gregorian Calendar, we can calculate the total frequency of the day of the week, which occurs on the 13^th day of the month. As a result, we can see, that the least number of weekdays on the 13^th day is 684 which refers to Thursday and Saturday. Next, for the 400-year period, we can observe Monday and Tuesday occurring exactly 685 times on the 13^th and Wednesday along with Sunday with a total number of 687. The most interesting thing is, that the Friday 13^th occurs 688 times in the 400 years period, which constitutes the biggest frequency!

A bit of information about the Gregorian Calendar I also wrote here.

References:

1. Bede the Venerable,1999, “Lunar Epacts”, The Reckoning of Time, Translated Texts for Historians, vol. 29, translated by Wallis, Faith, Liverpool: Liverpool University Press, p. 131, ISBN 0-85323-693-3
2. Derschowitz N., Reingold E.M., 2008, Calendrical calculations, Third Edition, Cambridge University Press, Cambridge.
3. Meeus J., 1997, Mathematical Astronomy Morsels, vol. 1, Willimann-Bell, ISBN-10. 0943396514
4. Meeus J., 2002, More Mathematical Astronomy Morsels, vol 2, Willmann-Bell, ISBN 0943396743
5. Richards, E. G.,2012, Calendars, (in:) Urban, S. E.; Seidelman, P. K. (eds.), Explanatory Supplement to the Astronomical Almanac, Mill Valley, CA: University Science Books, pp. 599–601, ISBN 978-1-891389-85-6

 

Links:

1. http://dates.gmarts.org/eastdate.htm
2. https://www.almanac.com/content/what-earth-epact
3. https://stason.org/TULARC/society/calendars/2-12-5-What-is-the-Epact.html
4. https://www.timeanddate.com/calendar/determining-easter-date.html
5. https://www.almanac.com/easter-paschal-full-moon

 

Wiki:

1. Date of Easter
2. Ecclesiastical full moon
3. Epact
4. Gregorian calendar